Rao's Spacing Test

Daniel J. Levitin and Gerald S. Russell
Department of Psychology, University of Oregon

To appear in:
Kotz, S. and Read, C. B. [Eds.], (1997).  Encyclopedia of Statistical Sciences, New York:  John Wiley & Sons.

This electronic Web version of the paper Copyright 1997 Daniel J. Levitin. Permission to make digital or hard copies of part or all of this work for personal or classroom use is granted with or without fee provided that copies are not made or distributed for profit or commercial advantage and that copies bear this notice and full citation on the first page. To copy otherwise,to republish, to post on services, or to redistribute to lists, requires specific permission and/or a fee.

Rao's Spacing Test is a useful and powerful statistic for testing uniformity of circular ("directional") data*. As with other circular statistics, Rao's Spacing Test is applicable for analysis of directional data, time series, similarity judgments involving musical pitch or color [5], phase relations in studies of movement [2] and spatial trends in geographical research [4]. In many cases, particularly with an underlying distribution that is multimodal, it is more powerful than the popular Rayleigh Test* and Kuiper's V Test*.

The statistic was first conceived in the doctoral dissertation of J. S. Rao [6] and is further described in Rao [7, 8] and Batschelet [1]. Rao's Spacing Test is based on the idea that if the underlying distribution is uniform, successive observations should be approximately evenly spaced, about 360/N apart. Large deviations from this distribution, resulting from unusually large spaces or unusually short spaces between observations, are evidence for directionality. It is related to the general class of linear statistical tests that are based on successive order statistics* and spacings*. A similar test for higher dimensions was independently described by Foutz [3], who was apparently unaware of Rao's previous work, and did not know the exact distribution.

Rao's test statistic U is defined as follows:

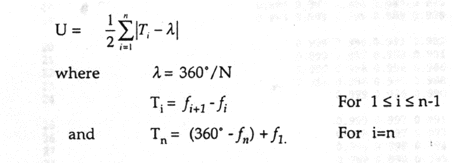
Because the sum of the positive deviations must equal the sum of the negative deviations, a simpler computational form eliminates absolute values, so that

U =

summed across positive deviations only.

The density function of U is known to be [8]:

for

where

Critical values of the U statistic were recently tabulated and published in Russell and Levitin [9].

Example.

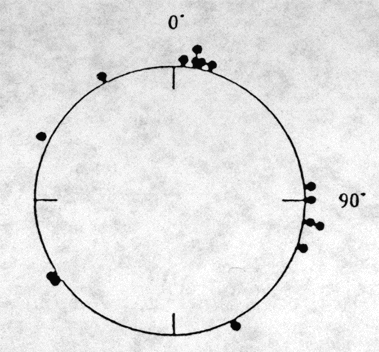
Suppose one wishes to know whether birth times at a hospital are uniformly distributed throughout the day. The alternative hypothesis is that there is a time (or times) when births are more frequent. Table 1 displays hypothetical data for delivery times collected across several days. These time series data form a continuous circular distribution, with times of day converted to angles around a circle, such that 12 midnight = 0°, 6 a.m. = 90°, and each minute = 360°/(24 hours * 60 minutes) = 0.25°. The distribution around the circle is shown graphically in Figure 1.

We find that {Pict 3}, and so {Pict 4}. From the tabled values for U(15) [9], p<.02, so we reject the hypothesis of uniformity, in favor of a hypothesis of modality or multi-modality. Close inspection of the data reveals bimodality, with peaks near 12:40 am and 6:38 am.

[Perhaps in an attempt to introduce circularity into his name, Jammalamadaka S. Rao currently publishes under the name S. Rao Jammalamadaka.]

References.

[1] Batschelet, E. (1981). Circular statistics for biology. Academic Press, London.

[2] Diedrich, F.J. and W.H. Warren, Jr. (1995). Why change gaits? Dynamics of the walk-run transition. Journal of Experimental Psychology: Human Perception & Performance, 21, 183-202.

[3] Foutz, R.V. (1980). A test for goodness-of-fit based on an empirical probability measure. Annals of statistics, 8, 989-1001.

[4] Lawson, A. (1988). On tests for spatial trend in nonhomogenous Poisson process. Journal of Applied Statistics, 15, 225-234.

[5] Levitin, D.J. (1994). Problems in applying the Kolmogorov-Smirnov Test: The need for circular statistics in psychology (Tech. Report #94-07). University of Oregon, Institute of Cognitive & Decision Sciences, Eugene, Oregon.

[6] Rao, J.S. (1969). Some contributions to the analysis of circular data. Ph.D. thesis, Indian Statistical Institute, Calcutta.

[7] Rao, J.S. (1972). Some variants of chi-square for testing uniformity on the circle. Zeitschrift für wahrscheinlichkeitstheorie und verwandte gebiete, 22, 33-44.

[8] Rao, J.S. (1976). Some tests based on arc-lengths for the circle. Sankhya: The Indian Journal of Statistics, Ser. B(4), 38, 329-338.

[9] Russell, G.S. and D.J. Levitin (1995). An expanded table of probability values for Rao's Spacing Test. Communications in statistics: Simulation and computation, 24, 879-888.

Bibliography.

[1] Puri, M.L., J.S. Rao, and Y. Yoon, A simple test for goodness-of-fit based on spacings with some efficiency comparisons, in Contributions to statistics, J. Jureùcková, Editor. 1979, D. Reidel, Dordrecht, Holland.

[2] Pyke, R. (1965). Spacings. Journal of the Royal Statistical Society Series B7, 395-449.

Suggested related entires: Circular Statistics, Directional Data Analysis, Goodness of fit, Order Statistics, Spacings, Uniform (Or Rectangular) Distributions.

i

Time

f

Ti

|Ti-l|

1

12:20 AM

5

5

19

2

12:40 AM

10

0

24

3

12:40 AM

10

2

22

4

12:48 AM

12

5

19

5

1:08 AM

17

68

44

6

5:40 AM

85

5

19

7

6:00 AM

90

9

15

8

6:36 AM

99

1

23

9

6:40 AM

100

10

14

10

7:20 AM

110

43

19

11

10:12 AM

153

80

56

12

3:32 PM

233

2

22

13

3:40 PM

235

61

37

14

7:44 PM

296

35

11

15

10:04 PM

331

34

10

n=15

Sigma=

354

Table 1. Calculation of the test statistic U for sample data given in text.

Arc length in this example = l =360°/n = 24°.

^(1/2)= 354/2 = 177

From tabled values, U(15) yields p<.02, so we reject the hypothesis of uniformity.

{Fig 1}

Figure 1. Time of day data (hypothetical) for births at a small hospital. The 24-hour clock times are converted to angular measures around a circle, with 1 minute = 0.25°. Rao's Spacing Test is used to test the null hypothesis that the birth times are uniformly distributed throughout a 24-hour day.

As of 8/1/96, send correspondence to Daniel J. Levitin, Interval Research Corporation, 1801C Page Mill Road, Palo Alto, CA 94304, (415) 842-6236, E-mail: levitin@interval.com

Go Back to Daniel Levitin's Home Page.